3. Laws of Physics in Inertial Frames

Any inertial frame is as good as another. If there are two inertial frames moving relative to each other, who is to say which one is moving and which one is at rest? Accordingly, all laws of physics must be the same in any inertial frame .

Consider an inertial frame A with coordinates (x,t) and another inertial frame B with coordinates (x',t') moving at constant velocity v in the x direction relative to A. What we are saying is that the laws of physics must be the same regardless of whether you are using the coordinates (x,t) of inertial frame A to describe the motion of an object, or the coordinates (x',t') of inertial frame B.

Now, (x,t) and (x',t') are related by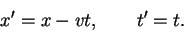

Here, we have assumed that the two clocks are set to agree with each other, and that the origin of the two frames are at the same point when t'=t=0. Using this relation, we can translate the observations of the observer in inertial frame A to that of the observer in inertial frame B, and vice versa. This translation prescription is known as the Galilei transformation.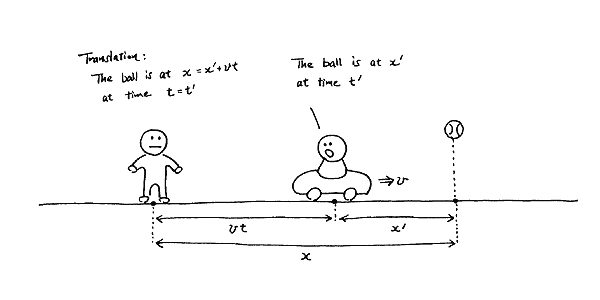

So the requirement that the laws of physics must be the same not matter which inertial frame is used to describe motions of objects can be restated as:

(This is one of the many expressions that physicists use to confuse the uninitiated.)

The following figures give a graphical representation of the Galilei transformation:

1. Understanding the Galilei transformation
2. Spacetime Diagram representation of the Galilei transformation

Back to Table of Contents

Previous  Next