This page serves as a location where the information pertaining to the shapes of single bubbles in the group are stored. This is meant to be a "launching point" for any aspiring researchers who intend to begin researching the mechanics of bubbles.
Most research in bubble mechanics is done using soap bubbles. This is due to the easy access to the materials necessary to make them as well as the manageable scale on which they exist. These bubbles consist of a soap dissolved into water, sometimes with added metal salts, to create a soap-water solution. When the bubble is not immersed in water, it is encapsulated by a thin film of the solution, whose high surface tension allows the surface to stay intact.
Thin films are generally separated into two
categories: dry films and wet films. Dry films have very little liquid between the interfaces of the films that
contain the bubble, and thus less surface flow inside the bubble. This makes it rather easy to make the
approximation that the films represent planes.
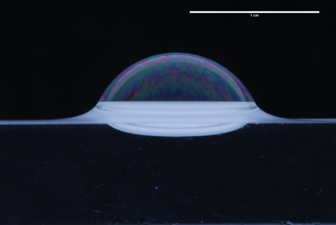
Wet films are more difficult to measure and simulate. This is because they contain fluid flow and other
effects caused by liquids. The most important and difficult aspect of this to handle is the capillary rise
of the liquid around the bubble, or the meniscus. This relies entirely on the surface tension of the liquid
that tries to flatten the top surface of the bubble.
The Laplace pressure as defined by the Young-Laplace Equation, is the difference in pressure between the inside and outside of a surface interface. It is defined as $$\Delta p = \gamma \left( \frac{1}{r_1} + \frac{1}{r_2}\right)$$ Where r1 and r2 are the two radii used to define the curvature of a two-dimensional surface, and γ is the surface tension of the film. If r1 and r2 are the same, as would be the case in a sphere-like section of the bubble, this reduces to $$\Delta p = \frac{2 \gamma}{r}$$
The standard Young-Laplace Equation looks as such:
$$\Delta p = \frac{2 \gamma}{r}$$
Where r is the local radius of curvature of the surface, γ is the surface tension,
and Δp is the change of pressure across the gas-liquid interface. However, this is not
applicable since the bubbles in question do not have a single liquid-gas
interface, but instead have 2 (one "inside" the bubble and another "outside" the bubble).
In the case of this thin-film barrier, the Young-Laplace Equation becomes
$$\Delta p = \frac{4 \gamma}{r}$$
This equation is part of what governs the shape and size of bubbles.
In addition to the Young-Laplace Equation, Plateau introduced laws that are necessary for equilibrium. These help to define the shapes of foams.
In a dry foam, the films can intersect only three at a time, and must do so at 120 degrees. The border at which these films intersect is known as the Plateau border. In two dimensions, this applies to the lines which define the cell boundaries.
In dry foams, there can be no more than 4 Plateau borders to meet at a junction. This implys that there are at most 6 surfaces that meet at a single junction. The angle at which the Plateau borders meet are always equal to $$\arccos\left(\frac{-1}{3}\right)$$
Where a Plateau border joins an adjacent film, the normal to that surface is the same on both sides of the intersection.
Foams and bubbles do not, in truth, have the capacity to exist in two dimensions. However, it is possible to create an environment in which they behave as though they existed only in two dimensions by "sandwiching" the foam between two sheets of glass. So long as the separation of the sheets of glass is much smaller than the cell size of the bubbles, the foam can be treated in two dimensions. In two dimensions, the foams still follow the Young-Laplace equation for thin films, but the cell walls are only circular arcs instead of a three dimensional curve. To simulate this, Voronoi Diagrams are often used.
Some people find difficulty in seeing the applications in this a field of research. Studying the shapes and mechanics of soap bubbles can be a very versatile endeavor. The mechanics of soap bubbles can be extended to determine the strength and reliability of solid foams, for safety or comfort purposes. They can also be seen in the grain structure of polycrystalline solids. An extreme application is modeling the superstructure as a foam, and using that model to predict energy transfers within that "foam".
1Stefan Hutzler and Denis Weaire The Physics of Foams, (Oxford University Press, New York, 1999).
Bubble Physics